Problem Set #2

1. Use the circle mnemonic (the Frost-Musulin mnemonic) to arrive at a display of orbital energies for the cycloheptatriene system. Populate the orbitals appropriately for the cation, and calculate its resonance energy. Do the same for the anion. The MO energies are: a+2b, a+0.62b,a+0.62b,a-0.44b,a-0.44b,a-1.80b,a-1.80b.

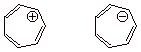
2. Using the concept of TS aromaticity/antiaromaticity, explain why carbocation rearrangments are so facile, and neither radicals nor carbanions rearrange at all. Supply an orbital picture, along with your explanation.

3. Again, using TS aromaticity/antiaromaticity, explain (with the assistance of orbital pictures), why S_N2 reactions prefer inversion over displacement. Do the same using FO (HOMO/LUMO) theory, taking into account orbital enlargement.

4. Use FO theory, with appropriate orbital pictures, to show why the Diels-Alder reaction of 1,3-butadiene (s-cis conformation) with ethylene is a facile reaction.

5. Employ the theory of TS aromaticity/antiaromaticity to predict the preferred mode of approach of carbene (CH₂) to ethylene.

6. Extra Credit: Explain what is meant by a Mobius conjugated system, and indicate such an orbital system in the TS for carbene addition to alkenes. (We will later see another example in the context of electrocyclic reactions).

Note: In the notes, I had suggested that you calculate the resonance energies of the cyclopentadienyl anion, radical, and cation, but I didn’t give you the MO energies. In case you are still interested in doing this as an exercise, the energies are: a+2b,a+0.62b,a+0.62b,a-1.62b,a-1.62b. Also, please be aware the HMO energies and coefficients can be obtained for virtually any stem from “Dictionary of Pi Electron Calculations” by Coulson and Streitweiser (A 2 Volume Set).